An introduction to Complex numbers

Exercise:

$z_1=2+5i$
$z_2=2-5i$

Find $z_1+z_2$ , $z_1-z_2$ , $z_1z_2$ and $z_1/z_2$

Solution:
We notice that $z_2=\overline{z_1}$

$z_1+z_2=2\times 2=4$
$z_1-z_2=2\times 5=10$
$z_1z_2=|z_1|=(\sqrt{2^2+5^2})^{2}=29$
$z_1/z_2=\frac{2+5i}{2-5i}=\frac{(2+5i)(2+5i)}{(2-5i)(2+5i)}=\frac{(4-25)+(10i+10i}{2^2-(5i)^{2}}=\frac{-21+20i}{29}=\frac{-21}{29}+\frac{20}{29}i$

Finally:
$z_1/z_2=\frac{-21}{29}+\frac{20}{29}i$

Evaluate:

$(1+i)^8$

Solution
Let $z=1+i$

We try to find the polar expression:
The modulus:
$|z|=\sqrt{1^2+1^2}=\sqrt{2}$

Now for the argument:
if $z=a+bi$ ,

$\cos \theta=\frac{a}{|z|}=\frac{1}{\sqrt{2}}$

$\sin \theta=\frac{b}{|z|}=\frac{1}{\sqrt{2}}$

We can see that $\theta=\frac{\pi}{4}$

Now we can write:
$z=|z|(\cos \theta+ i \sin \theta)=\sqrt{2}(\frac{1}{\sqrt{2}}+i \frac{1}{\sqrt{2}})$

Or even better:
$z=\sqrt{2}e^{i\frac{\pi}{4}}$

Now the fabulous De Moivre formula

$z^8=(2^{\frac{1}{2}})^8e^{i\frac{8\pi}{4}}$

$z^8=2^{\frac{8}{2}}e^{i2\pi}$ But remember: $-\pi<\theta \leq \pi$

So we get $\theta=2\pi-2\pi=0$

$z^8=2^{4}e^{i0}$

$z^8=2^{4}(\cos 0 + i \sin 0)$

$z^8=2^{4}(1+0i)$

$z^8=16$

Finally:

Answer: $(1+i)^8=16$

Problem:

Find the 8th roots of $z^8=1$

Solution:

$z^8=1$ , finding the 8 roots

Let $w=z^8=1$

$w=1+0i$

Modulus:

$|w|=1$

$\arg(w)=0$

We can write in polar form:

$w=e^{i(0+2k\pi}$

$z=e^{i(\frac{2k\pi}{8})}=e^{i(\frac{k\pi}{4})}$

$k=0,1,2,3,4,5,6,7$ here from $0$ to $n-1$

The roots:

$z_0=e^{i0}=(\cos 0+i\sin 0)=(1+0)=1$

$z_1=e^{i\frac{\pi}{4}}=(\cos \frac{\pi}{4}+ i \sin \frac{\pi}{4})=\frac{1}{\sqrt{2}}+\frac{1}{\sqrt{2}}i$

$z_2=e^{i\frac{2\pi}{4}}=e^{i\frac{\pi}{2}}=(\cos \frac{\pi}{2}+ i \sin \frac{\pi}{2})=0+1i=i$

$z_3=e^{i\frac{3\pi}{4}}=e^{i\frac{3\pi}{4}}=(\cos \frac{3\pi}{4}+ i \sin \frac{3\pi}{4})=-\frac{1}{\sqrt{2}}+\frac{1}{\sqrt{2}}i$

$z_4=e^{i\frac{4\pi}{4}}=e^{i\pi}=(\cos \pi+ i \sin \pi)=-1+0i=-1$

$z_5=e^{i\frac{5\pi}{4}}=e^{i\frac{-3\pi}{4}}=(\cos \frac{-3\pi}{4}+ i \sin \frac{-3\pi}{4})=-\frac{1}{\sqrt{2}}-\frac{1}{\sqrt{2}}i$

$z_6=e^{i\frac{6\pi}{4}}=e^{i\frac{-2\pi}{4}}=e^{i\frac{-\pi}{2}}=(\cos \frac{-\pi}{2}+ i \sin \frac{-\pi}{2})=0-1i=-i$

$z_7=e^{i\frac{7\pi}{4}}=e^{i\frac{-\pi}{4}}=(\cos \frac{-\pi}{4}+ i \sin \frac{-\pi}{4})=\frac{1}{\sqrt{2}}-\frac{1}{\sqrt{2}}i$

Finally:

Answer: $1;\frac{1}{\sqrt{2}}+\frac{1}{\sqrt{2}}i;i;-\frac{1}{\sqrt{2}}+\frac{1}{\sqrt{2}}i;-1;-\frac{1}{\sqrt{2}}-\frac{1}{\sqrt{2}}i;-i;\frac{1}{\sqrt{2}}-\frac{1}{\sqrt{2}}i$

Solve for $z$

$z^2-(3+i)z+4+3i=0$

Solution

$z^2-(3+i)z+4+3i=0$

$\Delta=(-(3+i))^2-4(4+3i)$

$\Delta=(9+6i+i^2)-(16+12i)$

$\Delta=9+6i-1-16-12i$

$\Delta=-8-6i$

Our challenge is to find another complex number, the $\sqrt{\Delta}$

Let $w^2=-8-6i$

The modulus:

$|w|=\sqrt{(-8)^2+(-6)^2}=\sqrt{100}=10$

Now:

$w^2=10(-\frac{8}{10}-\frac{6}{10}i)=10(-\frac{4}{5}-\frac{3}{5}i)$

Base angle:

$\cos \theta=\frac{4}{5}\Leftarrow \theta=36.8699 ^\circ$

From our unit circle, the with both sine and cosine <0 is base angle plus 180 degrees.

$\arg(w^2)=216.8699$

However, our notation $-\pi<\arg(z)\leq \pi$ makes it $\theta=-143.1301 ^{\circ}$

Now:

$w^2=10e^{-i143.1301}$

$w=\sqrt{10}e^{-\frac{i143.1301}{2}}=\sqrt{10}e^{-i71.56505}$

$w=\sqrt{10}(\cos (-71.56505^{\circ})+\sin (-71.56505^{\circ})i)$

$w=\sqrt{\Delta}=1-3i$

$z_1=\frac{-(-(3+i))+(1-3i)}{2}=\frac{3+i+1-3i}{2}=\frac{4-2i}{2}=2-i$

$z_2=\frac{-(-(3+i))-(1-3i)}{2}=\frac{3+i-1+3i}{2}=\frac{2+4i}{2}=1+2i$

Finally:

Answer: $(z_1=2-i; \; z_2=1+2i)$

Problem:

Solve for $z$

$z^3=-2+2i$

Solution

$z^3=-2+2i$

Let $w=z^3=-2+2i$

Modulus:

$|w|=\sqrt{2^2+2^2}=\sqrt{8}$ Please note that $\sqrt{8}=2\sqrt{2}$

Now the arg(w)

$w=\sqrt{8}(-\frac{2}{2\sqrt{2}}+i\frac{2}{2\sqrt{2}})=\sqrt{8}(-\frac{1}{\sqrt{2}}+i\frac{1}{\sqrt{2}})$

From the unit circle:

The base angle here is $\frac{\pi}{4}$

Now a combination of the trigonometric functions give $\arg(w)=\frac{3\pi}{4}$

We need to find 3 roots with $k=0,1,2$

The notation becomes:

$w=z^3=\sqrt{8}e^{i\frac{3\pi}{4}}$

Now:

$z=((8)^{\frac{1}{2}})^{\frac{1}{3}}e^{i(\frac{\frac{3\pi}{4}+2k\pi}{3})}=\sqrt{2}e^{i(\frac{\pi}{4}+\frac{2k\pi}{3})}$

The roots:

$z_0=\sqrt{2}e^{i\frac{\pi}{4}}=\sqrt{2}(\cos \frac{\pi}{4}+ i \sin \frac{\pi}{4})=\frac{\sqrt{2}}{\sqrt{2}}+\frac{\sqrt{2}}{\sqrt{2}}i=1+i$

$z_0=1+i$

$z_1=\sqrt{2}e^{i(\frac{\pi}{4}+\frac{2\pi}{3})}$

Some future formulas:

$\cos (\frac{\pi}{4}+\frac{2\pi}{3})=\cos (\frac{\pi}{4})\cos \frac{2\pi}{3}-\sin (\frac{\pi}{4})\sin \frac{2\pi}{3}=\frac{\sqrt{2}}{2}\cdot (\frac{-1}{2})-\frac{\sqrt{2}}{2}\cdot (\frac{\sqrt{3}}{2})=\frac{-\sqrt{2}-\sqrt{2}\sqrt{3}}{4}$

$\sin (\frac{\pi}{4}+\frac{2\pi}{3})=\sin (\frac{\pi}{4})\cos \frac{2\pi}{3}+\cos (\frac{\pi}{4})\sin \frac{2\pi}{3}=\frac{\sqrt{2}}{2}\cdot (\frac{-1}{2})+\frac{\sqrt{2}}{2}\cdot (\frac{\sqrt{3}}{2})=\frac{-\sqrt{2}+\sqrt{2}\sqrt{3}}{4}$

$z_1=\sqrt{2}(\cos (\frac{\pi}{4}+\frac{2\pi}{3})+i \sin (\frac{\pi}{4}+\frac{2\pi}{3})=\sqrt{2}((\frac{-\sqrt{2}-\sqrt{2}\sqrt{3}}{4})+i(\frac{-\sqrt{2}+\sqrt{2}\sqrt{3}}{4}))=(\frac{-1-\sqrt{3}}{2})+i(\frac{-1+\sqrt{3}}{2})$

$z_1=(\frac{-1-\sqrt{3}}{2})+i(\frac{-1+\sqrt{3}}{2})$

For $z_2$ we use the transformation formulas and we get:

$z_2=\sqrt{2}e^{i(\frac{\pi}{4}+\frac{4\pi}{3})}=\sqrt{2}e^{i(\frac{-5\pi}{2})}$

$z_2=(\frac{\sqrt{3}-1}{2})+i(\frac{-1-\sqrt{3}}{2})$

Problem:
Evaluate $(1+i)^{4}$

Solution

This can be done 2 ways

The peasant way,
Use binomial and note that this can be lengthy if the exponent is high

$(1+i)^4=1+4i+6i^2+4i^3+i^4=1+4i-6-4i+1=-4$

The poet way:
Use complex numbers operations. Always try this way.
$z=1+i$
Modulus:
$|z|=\sqrt{1^2+1^2}=\sqrt{2}$

$z=1+i=|z|(\cos \theta +i \sin \theta)=\sqrt{2}(\frac{1}{\sqrt{2}}+i\frac{1}{\sqrt{2}})$

The base angle: $\theta=\frac{\pi}{4}$ and is also the $\arg(z)$
$z=\sqrt{2}e^{i\frac{\pi}{4}}$
$z^4=(2)^{\frac{1}{2}\cdot 4}e^{i\frac{4\pi}{4}}$

$z^4=(2)^{2}e^{i\pi}$

$z^4=4(cos \pi+ i\sin \pi)=4(-1+ i\cdot 0)=-4$

Finally:
Answer: $(1+i)^4=-4$

Problem:
Evaluate $(1+2i)(3-i)$

Solution

$(1+2i)(3-i)=1\cdot 3+1\cdot(-i)+(2i)\cdot 3-(2i)\cdot (-i)=3-i+6i-2i^2=5+5i$

The second method, more efficient for multiple comple numbers.

$|1+2i|=\sqrt{5}$
$1+2i=\sqrt{5}(\frac{1}{\sqrt{5}}+i\frac{2}{\sqrt{5}})$

Base angle
$\theta_1=\cos ^{-1}\frac{1}{\sqrt{5}}=63.435^{\circ}$
With the $sine$ showing $>0$ , we are in the first quadrant of the unit circle.
So, $\arg(1+2i)=63.435^{\circ}$

$|3-i|=\sqrt{10}$
$3-i=\sqrt{10}(\frac{3}{\sqrt{10}}-i\frac{1}{\sqrt{10}})$

Base angle
$\theta_1=\cos ^{-1}\frac{3}{\sqrt{10}}=18.435^{\circ}$
With the $sine$ showing $<0$ , we are in the fourth quadrant of the unit circle.
So, $\arg(3-i)=-18.435^{\circ}$

The product:

$(1+2i)(3-i)=\sqrt{5} \sqrt{10}e^{i(63.435^{\circ}+(-18.435^{\circ}))}$

$(1+2i)(3-i)=\sqrt{50}e^{i45^{\circ}}=\sqrt{50}(\cos 45^{\circ} +i \sin 45^{\circ})=5+5i$

Answer: $(1+2i)(3-i)=5+5i$

Problem:
Evaluate $\frac{1+2i}{3-i}$

Solution

Two methods:

The first method
$\frac{1+2i}{3-i}=\frac{(1+2i)(3+i)}{(3-i)(3+i)}=\frac{3+i+6i-2}{9+1}=\frac{1}{10}+i\frac{7}{10}$

The second method, more efficient for multiple complex numbers.

$|1+2i|=\sqrt{5}$
$1+2i=\sqrt{5}(\frac{1}{\sqrt{5}}+i\frac{2}{\sqrt{5}})$

Base angle
$\theta_1=\cos ^{-1}\frac{1}{\sqrt{5}}=63.435^{\circ}$
With the $sine$ showing $>0$ , we are in the first quadrant of the unit circle.
So, $\arg(1+2i)=63.435^{\circ}$

$|3-i|=\sqrt{10}$
$3-i=\sqrt{10}(\frac{3}{\sqrt{10}}-i\frac{1}{\sqrt{10}})$

Base angle
$\theta_1=\cos ^{-1}\frac{3}{\sqrt{10}}=18.435^{\circ}$
With the $sine$ showing $<0$ , we are in the fourth quadrant of the unit circle.
So, $\arg(3-i)=-18.435^{\circ}$

$\frac{1+2i}{3-i}=\frac{\sqrt{5}}{\sqrt{10}}e^{i(63.435^{\circ}-(-18.435^{\circ}))}$
$\frac{1+2i}{3-i}=\frac{\sqrt{5}}{\sqrt{10}}e^{i81.870^{\circ}}=\frac{1}{10}+i \frac{7}{10}$

Answer: $\frac{1+2i}{3-i}=\frac{1}{10}+i \frac{7}{10}$

Problem:
Evaluate $z=\left(\frac{1-i}{\sqrt{2}}\right)^{-6}$

Solution

$z=\left(\frac{1-i}{\sqrt{2}}\right)^{-6}$

For the conjugate we know:

$\frac{1}{w}=\frac{\overline{w}}{|w|}$

Let $\overline{w}=\frac{1-i}{\sqrt{2}}=\frac{1}{\sqrt{2}}-\frac{i}{\sqrt{2}}$

The values of the angle are exposed

We know that the modulus $|w|=|\overline{w}|$

$|w|=\sqrt{(\frac{1}{\sqrt{}2})^{2}+(\frac{1}{\sqrt{}2})^{2}}=1$

That means:

$w=\frac{1}{\sqrt{2}}+\frac{i}{\sqrt{2}}$

$\left(\overline{w}\right)^{-6}=\left(\frac{w}{|w|^2}\right)^6$

This brings home:

$z=\left(\frac{1}{\sqrt{2}}+\frac{i}{\sqrt{2}}\right)^6$

Regular calculation:

$w=\frac{1}{\sqrt{2}}+\frac{i}{\sqrt{2}}$

Modulus $|w|=1$ and base angle: $\arg(w)=\frac{\pi}{4}$ since we are in first quadrant.

$z=e^{i\frac{6\pi}{4}}=e^{i\frac{3\pi}{2}}$ But remember $-\pi<\theta \leq \pi$

That means:

$z=e^{-i\frac{\pi}{2}}=\cos \frac{-\pi}{2}+i \sin \frac{-\pi}{2}=0-i=-i$

Finally

Answer: $z=-i$

Exercise 27C (From EARL):

Let $\xi=cos \frac{2\pi}{5}+i \sin \frac{2\pi}{5}$

Show that $\xi^5=1$ and deduce that $1+\xi+\xi^2+\xi^3+\xi^4=0$

Find the quadratic equation with the roots $\xi+\xi^4$ and $\xi^2+\xi^3$ .

Hence show that $\cos \frac{2\pi}{5}=\frac{\sqrt{5}-1}{4}$

Our Approach and solution:

In the polar coordinates:

$\xi=e^{i\frac{2\pi}{5}}$

$\xi^5=1 \Rightarrow \xi^5-1=0$

We know that:

$x^n-1=(x-1)(1+x+x^2+\cdots+x^{n-1})$

This means:

$\xi^{5}-1=(\xi-1)(1+\xi+\xi^2+\xi^3+\xi^4)$

To get 5 roots reals and complex, both factors must be equal to $0$

We get:

$(1+\xi+\xi^2+\xi^3+\xi^4)=0$

To find the roots of the quadratic, let’s evaluate each element:

With in mind the argument $-\pi<\theta \leq \pi$

$\xi=e^{i\frac{2\pi}{5}}$

$\xi^{2}=e^{i\frac{4\pi}{5}}$

$\xi^{3}=e^{i\frac{6\pi}{5}}=e^{i\frac{-4\pi}{5}}$

$\xi^{4}=e^{i\frac{8\pi}{5}}=e^{i\frac{-2\pi}{5}}$

The roots are:

$\xi+\xi^4$ and $\xi^2+\xi^3$

The product:

$(\xi+\xi^4)(\xi^2+\xi^3)=(e^{i\frac{2\pi}{5}}+e^{i\frac{-2\pi}{5}})(e^{i\frac{4\pi}{5}}+e^{i\frac{-4\pi}{5}}$

$(\xi+\xi^4)(\xi^2+\xi^3)=e^{i\frac{2\pi}{5}}+e^{i\frac{4\pi}{5}}+e^{i\frac{-4\pi}{5}}+e^{i\frac{-2\pi}{5}}$

This means we can change the following:

$(1+\xi+\xi^2+\xi^3+\xi^4)=0$ to:

$e^{i\frac{2\pi}{5}}+e^{i\frac{4\pi}{5}}+e^{i\frac{-4\pi}{5}}+e^{i\frac{-2\pi}{5}}=0$

$2\cos \frac{2\pi}{5}+2\cos \frac{4\pi}{5}+1=0$

When we convert:

$2\cos \frac{2\pi}{5}+4\cos^{2} \frac{2\pi}{5}-2+1=0$

$4\cos^{2} \frac{2\pi}{5}+2\cos \frac{2\pi}{5}-1=0$

Let $\cos \frac{2\pi}{5}=x$

$4x^{2}+2x-1=0$

$\Delta=2^2-4(4)(-1)=20$
$\sqrt{\Delta}=\sqrt{20}=2\sqrt{5}$

Before we go further, please note that $x>0$ since our angle is the first quadrant.

$x=\frac{-2+2\sqrt{5}}{8}=\frac{\sqrt{5}-1}{4}$

Finally:

Answer: $\cos \frac{2\pi}{5}=\frac{\sqrt{5}-1}{4}$

Exercise 28C (EARL)

Determine the modulus and argument of the following complex numbers:

$i+i$ and $\sqrt{3}+i$ .

Calculate $\frac{1+i}{\sqrt{3}+i}$ using polar coordinates and $a+bi$ form.

Deduce the $\sin \frac{\pi}{12}$ and $\cos \frac{\pi}{12}$ .

Our Solution:

$z_1=1+i$

modulus:

$|z_1|=\sqrt{1+1}=\sqrt{2}$

$z_1=\sqrt{2}(\frac{1}{\sqrt{2}}+i\frac{1}{\sqrt{2}})$

$arg(z_1)=\frac{\pi}{4}$

$z_1=\sqrt{2}e^{i\frac{\pi}{4}}$

$z_2=\sqrt{3}+i$

modulus:

$|z_2|=\sqrt{3+1}=\sqrt{4}=2$

$z_2=2(\frac{\sqrt{3}}{2}+i\frac{1}{2})$

$arg(z_2)=\frac{\pi}{3}$

$z_2=\sqrt{2}e^{i\frac{\pi}{3}}$

Now Calculating $\frac{1+i}{\sqrt{3}+i}$

$\frac{1+i}{\sqrt{3}+i}=\frac{(1+i)(\sqrt{3}-i)}{(\sqrt{3}+i)(\sqrt{3}-i)}$

$\frac{1+i}{\sqrt{3}+i}=\frac{\sqrt{3}+1-i+\sqrt{3}i}{3+1}=\frac{\sqrt{3}+1}{4}+i\frac{\sqrt{3}-1}{4}$

In polar form:

$\frac{z_1}{z_2}=\frac{\sqrt{2}}{2}e^{i(\frac{\pi}{4}-\frac{\pi}{3})}=\frac{\sqrt{2}}{2}e^{-i\frac{\pi}{12}}$

$\frac{z_1}{z_2}=\frac{\sqrt{2}}{2}(cos (-\frac{\pi}{12})+i \sin (-\frac{\pi}{12}))$

$\frac{z_1}{z_2}=\frac{\sqrt{2}}{2}(cos (-\frac{\pi}{12})+i\frac{\sqrt{2}}{2}\sin (-\frac{\pi}{12}))$

Now we can say:

$\frac{\sqrt{2}}{2}(cos (-\frac{\pi}{12}))=\frac{\sqrt{3}+1}{4}$

$cos \frac{\pi}{12}=\frac{2}{\sqrt{2}}\cdot \frac{\sqrt{3}+1}{4}=\frac{\sqrt{3}+1}{2\sqrt{2}}$

$\frac{\sqrt{2}}{2}\sin (-\frac{\pi}{12})=\frac{\sqrt{3}-1}{4}$

$\frac{\sqrt{2}}{2}\sin (\frac{\pi}{12})=\frac{1-\sqrt{3}}{4}$

$\sin \frac{\pi}{12}=\frac{2}{\sqrt{2}}\cdot \frac{1-\sqrt{3}}{4}=\frac{1-\sqrt{3}}{2\sqrt{2}}$

Finally

Answer: $\sin \frac{\pi}{12}=\frac{1-\sqrt{3}}{2\sqrt{2}}$ and $\cos \frac{\pi}{12}=\frac{\sqrt{3}+1}{2\sqrt{2}}$

Exercise 30 B (EARL):

Using the 7th roots of -1 show that:

$\cos \frac{\pi}{7}+\cos \frac{3\pi}{7}+\cos \frac{7\pi}{7}=\frac{1}{2}$

Use a similar process to evaluate:

$\cos \frac{2\pi}{7}+\cos \frac{4\pi}{7}+\cos \frac{6\pi}{7}$

Solution:

$w=z^7=-1$

Modulus:
$|w|=1$

Argument:
$\cos \theta=-1$ and $\sin \theta=0 \Leftarrow \theta=\pi$

This means $\arg(w)=\pi$

$w=e^{i\pi}$

The 7th roots:

$z=e^{i(\frac{\pi+2k\pi}{7})}$ with $k=0,1,2,3,4,5,6$

$z=e^{i(\frac{\pi}{7}+{2k\pi}{7})}$

$z_1=e^{i(\frac{\pi}{7}+{0\times 2\pi}{7})}=e^{i\frac{\pi}{7}}$

$z_2=e^{i(\frac{\pi}{7}+{1\times 2\pi}{7})}=e^{i\frac{3\pi}{7}}$

$z_3=e^{i(\frac{\pi}{7}+{2\times 2\pi}{7})}=e^{i\frac{5\pi}{7}}$

$z_4=e^{i(\frac{\pi}{7}+{3\times 2\pi}{7})}=e^{i\pi}$

$z_5=e^{i(\frac{\pi}{7}+{4\times 2\pi}{7})}=e^{-i\frac{5\pi}{7}}$

$z_6=e^{i(\frac{\pi}{7}+{5\times 2\pi}{7})}=e^{-i\frac{3\pi}{7}}$

$z_7=e^{i(\frac{\pi}{7}+{6\times 2\pi}{7})}=e^{-i\frac{\pi}{7}}$

Sum of roots is $0$

$2\cos \frac{\pi}{7}+2\cos \frac{3\pi}{7}+2\cos \frac{7\pi}{7}-1=0$

This yields:

$\cos \frac{\pi}{7}+\cos \frac{3\pi}{7}+2\cos \frac{7\pi}{7}=\frac{1}{2}$

The same way, if we let:

$z^7=1$ we get the following:

$\cos \frac{2\pi}{7}+\cos \frac{4\pi}{7}+\cos \frac{6\pi}{7}=-\frac{1}{2}$

Finally:

$\cos \frac{\pi}{7}+\cos \frac{3\pi}{7}+2\cos \frac{7\pi}{7}=\frac{1}{2}$

$\cos \frac{2\pi}{7}+\cos \frac{4\pi}{7}+\cos \frac{6\pi}{7}=-\frac{1}{2}$

Solve for $z$

$z^2+\frac{1}{2}z+\frac{1}{4}=0$

[accordion hideSpeed=”300″ showSpeed=”400″]

[item title=”Click here to see the solution of: $z^2+\frac{1}{2}z+\frac{1}{4}=0$ “]

Solution:

$z^2+\frac{1}{2}z+\frac{1}{4}=0$

$\Delta=\left(\frac{1}{2}\right)^{2}-4\times 1 \times\frac{1}{4}=-\frac{3}{4}=i^{2}\frac{3}{4}$

$\sqrt{\Delta}=\frac{3}{2}i$

Finally:

Answer: $z=-\frac{1}{4}\pm \frac{\sqrt{3}}{4}i$

[/item] [/accordion]

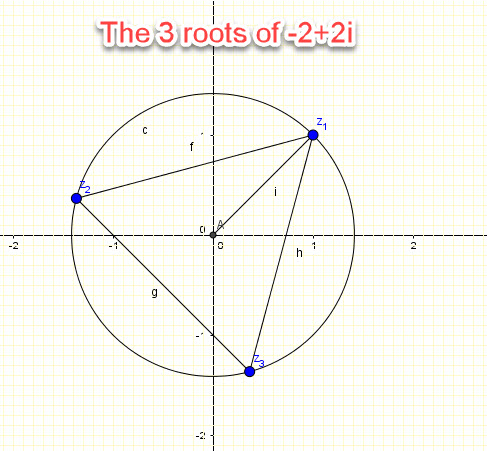
Solve $z^3=i$

Draw the roots and calculate the area of the triangle formed by the three roots.

Solution:

Modulus:

$|z^3|=1$

$Z^3=0+i$

$z^3=\cos \frac{\pi}{2}+i \sin \frac{\pi}{2}$

$\arg(z^3)=\frac{\pi}{2}$

$z^3=e^{i\frac{\pi}{2}}$

$z=e^{i(\frac{\frac{\pi}{2}+3k\pi}{3})}=e^{i(\frac{\pi}{6}+\frac{2k\pi}{3})}$

$z_1=e^{i\frac{\pi}{6}}= \cos \frac{\pi}{6}+ i \sin \frac{\pi}{6}=\frac{\sqrt{3}}{2}+ \frac{1}{2}i$

$z_2=e^{i(\frac{\pi}{6}+\frac{2\pi}{3})}=e^{i\frac{5\pi}{6}}= \cos \frac{5\pi}{6}+ i \sin \frac{5\pi}{6}=-\frac{\sqrt{3}}{2}+ \frac{1}{2}i$

$z_3=e^{i(\frac{\pi}{6}+\frac{4\pi}{3})}=e^{i\frac{-\pi}{2}}=\cos \frac{-\pi}{2}+ i \sin \frac{-\pi}{2}=0-i=-i$

Finally:

Answers: $z=(\frac{\sqrt{3}}{2}+ \frac{1}{2}i,\;-\frac{\sqrt{3}}{2}+ \frac{1}{2}i,\;-i)$

Write in $a+bi$ form:

$z=e^{\pi+i}$

Solution:

$z=e^{\pi}e^{i\cdot 1}$

Please note $1$ is 1 radian or $\frac{180}{\pi}$ in degrees.

So:

$z=e^{\pi}(\cos \frac{180}{\pi}+\sin \frac{180}{\pi}i)=12.50+19.47i$

Finally:

Answer: $z=12.50+19.47i$

1 2 3 4 5 6 7

Post Views: 572

Be the first to comment

Leave a Reply Cancel reply