Integration Techniques, multiple pages

February 29, 2020 Mouctar Calculus 0

2.Integration by parts

This is the technique of transforming one integral into another that is easier to evaluate.

The integration by parts comes from the following derivatives formula:

(uv)'=u'v+uv'

This can be written:

uv'=(uv)'-u'v

Taking the indefinite integral of both side we get:

\int udv=\int d(uv)-\int vdu

Or simply:

{\displaystyle \int u\, dv =uv-\int v\, du}

We must make an adequate choice in order not to get a more complicated integrated.

One of the favorite examples is:

Evaluate {\displaystyle \int \ln x\, dx}

Let u=\ln x \Rightarrow du=\frac{dx}{x}

dv=dx \Rightarrow v=x

 {\displaystyle \int \ln x\, dx=x \ln x-\int x\cdot \frac{dx}{x}=x \ln x-x+C}

Problem 13
Evaluate: {\displaystyle \int xe^{x}\, dx}

Let u=x \Rightarrow du=dx

dv=e^{x}dx \Rightarrow v=e^{x}

We get:

(1)   \begin{equation*} \begin{split} \displaystyle \int xe^{x}\, dx}&={\displaystyle xe^{x}- \int e^{x}\, dx}\\ &={\displaystyle xe^{x}- e^{x}+C} \end{split} \end{equation*}

Finally:

{\displaystyle \int xe^{x}\, dx=(x-1)e^{x}+C}

Problem 14
Evaluate: {\displaystyle \int e^{2x} \sin 3x\, dx}

Let u=\sin3x \Rightarrow du=3\cos 3x dx

dv=e^{2x}dx \Rightarrow v=\frac{1}{2}e^{2x}

We write:

(2)   \begin{equation*} \begin{split} \displaystyle \int e^{2x} \sin 3x\, dx}& = {\displaystyle \frac{1}{2} \sin (3x) e^{2x}-\frac{1}{2}\int e^{2x} 3\cos 3x\, dx}\\ &={\displaystyle \frac{1}{2} \sin (3x) e^{2x}-\frac{3}{2}\int e^{2x} \cos 3x\, dx} \end{split} \end{equation*}

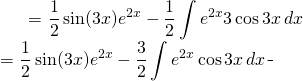
We resume the process for the second part of the integral:

Let u=\cos3x \Rightarrow du=-3\sin 3x dx

dv=e^{2x}dx \Rightarrow v=\frac{1}{2}e^{2x}

(3)   \begin{equation*} \begin{split} \displaystyle \int e^{2x} \sin 3x\, dx}&={\displaystyle \frac{1}{2} \sin (3x) e^{2x}-\frac{3}{2}[\frac{1}{2} \cos (3x) e^{2x}+\frac{3}{2}\int e^{2x} \sin 3x\, dx]}\\ &= {\displaystyle \frac{1}{2} \sin (3x) e^{2x}-\frac{3}{4}\cos (3x) e^{2x}-\frac{9}{4}\int e^{2x} \sin 3x\, dx \end{split} \end{equation*}

We are back to a fraction of the original:

{\displaystyle (1+\frac{9}{4})\int e^{2x} \sin 3x\, dx}={\displaystyle \frac{1}{2} \sin (3x) e^{2x}-\frac{3}{4}\cos (3x) e^{2x}+C}

{\displaystyle \frac{13}{4}\int e^{2x} \sin 3x\, dx}={\displaystyle \frac{1}{2} \sin (3x) e^{2x}-\frac{3}{4}\cos (3x) e^{2x}+C}

{\displaystyle \int e^{2x} \sin 3x\, dx}={\displaystyle \frac{2}{13} \sin (3x) e^{2x}-\frac{3}{13}\cos (3x) e^{2x}+C}

{\displaystyle \int e^{2x} \sin 3x\, dx}={\displaystyle \frac{e^{2x}}{13} (2\sin 3x -3\cos 3x )+C}

Finally:

{\displaystyle \int e^{2x} \sin 3x\, dx}={\displaystyle \frac{e^{2x}}{13} (2\sin 3x -3\cos 3x )+C}

Problem 15
Evaluate: {\displaystyle \int \sin^{-1} x\, dx}

Let u=\sin^{-1} x \Rightarrow du=\frac{dx}{\sqrt{1-x^{2}}}

dv=dx \Rightarrow v=x

We write:

(4)   \begin{equation*} \begin{split} \displaystyle \int \sin^{-1} x\, dx}&={\displaystyle x sin^{-1} x- \int \frac{x}{\sqrt{1-x^{2}}} \, dx}\\ &={\displaystyle x sin^{-1} x+ \frac{1}{2} \int \frac{-2x}{\sqrt{1-x^{2}}}}\\ &={\displaystyle x sin^{-1} x-\sqrt{1-x^{2}}+C \end{split} \end{equation*}

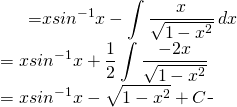
Finally:

{\displaystyle \int \sin^{-1} x\, dx}={\displaystyle x sin^{-1} x-\sqrt{1-x^{2}}+C}

Problem 16
Evaluate: {\displaystyle \int e^{x} \cos x\, dx}

Let u=e^{x} \Rightarrow du=e^{x}\, dx

dv=\cos x\,dx \Rightarrow v=\sin x

We write:

{\displaystyle \int e^{x} \cos x\, dx}={\displaystyle e^{x} \sin x - \int e^{x} \sin x\, dx}

We resume:

Let u=e^{x} \Rightarrow du=e^{x}\, dx

dv=\sin x\,dx \Rightarrow v=-\cos x

Back to the original equation:

{\displaystyle \int e^{x} \cos x\, dx}={\displaystyle e^{x} \sin x -[-e^{x} \cos x + \int e^{x} \cos x\, dx]}

{\displaystyle \int e^{x} \cos x\, dx}={\displaystyle e^{x} \sin x + e^{x} \cos x - \int e^{x} \cos x\, dx}

{\displaystyle 2\int e^{x} \cos x\, dx}={\displaystyle e^{x} \sin x + e^{x} \cos x + C}

{\displaystyle \int e^{x} \cos x\, dx}={\displaystyle \frac{e^{x}}{2}( \sin x + \cos x )+ C}

Finally:

{\displaystyle \int e^{x} \cos x\, dx}={\displaystyle \frac{e^{x}}{2}( \sin x + \cos x )+ C}

Problem 17- Special Case
Evaluate: {\displaystyle \int \sec^{n} x \, dx}

When n is a large natural number,we decrease n by 2 to case a situation where we have to integrate \sec^{2} x

We proceed as follows:

Let u=\sec^{n-2}x \Rightarrow du=(n-2)\sec^{n-2} x \tan x \, dx

dv=\sec^{2} x \,dx \Rightarrow v=\tan x

We get:

{\displaystyle \int \sec^{n} x \, dx} ={\displaystyle \sec^{n-2}x \tan x- (n-2) \int \sec^{n-2} x \tan^{2} x \, dx}

Or:

{\displaystyle \int \sec^{n} x \, dx} ={\displaystyle \sec^{n-2}x \tan x- (n-2) \int \sec^{n-2} x (\sec^{2} x-1) \, dx}

{\displaystyle \int \sec^{n} x \, dx} ={\displaystyle \sec^{n-2}x \tan x- (n-2) \int \sec^{n} x \, dx+ (n-2) \int \sec^{n-2} x \, dx}

We find the original integral in the right part. We group and we get:

{\displaystyle (n-2+1)\int \sec^{n} x \, dx} ={\displaystyle \sec^{n-2}x \tan x+ (n-2) \int \sec^{n-2} x \, dx}

{\displaystyle (n-1)\int \sec^{n} x \, dx} ={\displaystyle \sec^{n-2}x \tan x+ (n-2) \int \sec^{n-2} x \, dx}

We get:

{\displaystyle \int \sec^{n} x \, dx} ={\displaystyle \frac{ \sec^{n-2}x \tan x}{n-1}+\frac{n-2}{n-1} \int \sec^{n-2} x\, dx}

This is very useful when combined with our know formula:

{\displaystyle \int \sec x \, dx} ={\displaystyle \ln |\sec x+ \tan x | +C}

Problem 18
Evaluate: {\displaystyle \int \sec^{5} x \, dx}

Let u=\sec^{5-2}x \Rightarrow du=(5-2)\sec^{5-2} x \tan x \, dx

Or:

u=\sec^{3}x \Rightarrow du=3\sec^{3} x \tan x \, dx

dv=\sec^{2} x \,dx \Rightarrow v=\tan x

We get:

{\displaystyle \int \sec^{5} x \, dx} = {\displaystyle \frac{1}{4}\sec^{3}x \tan x +\frac{3}{4} \int \sec^{3} x \, dx}

Now, just for fun

{\displaystyle \int \sec^{3} x \, dx} ={\displaystyle \int \sec^{2} x \sec x \, dx}

Let u=\sec x \Rightarrow du=\sec x \tan x \, dx

dv=\sec^{2} x \,dx \Rightarrow v=\tan x

{\displaystyle \int \sec^{3} x \, dx} ={\displaystyle \sec x \tan x-\int \sec x \tan x \tan x \,dx}

{\displaystyle \int \sec^{3} x \, dx}={\displaystyle \sec x \tan x-\int \sec x \tan^{2} x \,dx}

{\displaystyle \int \sec^{3} x \, dx}={\displaystyle \sec x \tan x-\int \sec x (\sec^{2} x-1) \,dx}

{\displaystyle \int \sec^{3} x \, dx}={\displaystyle \sec x \tan x-\int \sec^{3} x+ \int \sec x \,dx}

{\displaystyle 2\int \sec^{3} x \, dx}={\displaystyle \sec x \tan x+ \int \sec x \,dx}

{\displaystyle 2\int \sec^{3} x \, dx}={\displaystyle \sec x \tan x+ \ln| \sec x + tan x |+C}

{\displaystyle \int \sec^{3} x \, dx}={\displaystyle \frac{1}{2}(\sec x \tan x+ \ln| \sec x + tan x |)+C}

Back to the original equation:

{\displaystyle \int \sec^{5} x \, dx} = {\displaystyle \frac{1}{4}\sec^{3}x \tan x +\frac{3}{4}\cdot \frac{1}{2}(\sec x \tan x+ \ln| \sec x + tan x |)+C}

{\displaystyle \int \sec^{5} x \, dx} = {\displaystyle \frac{1}{4}\sec^{3}x \tan x +\frac{3}{8}(\sec x \tan x+ \ln| \sec x + tan x |)+C}

Finally:

{\displaystyle \int \sec^{5} x \, dx} = {\displaystyle \frac{1}{4}\sec^{3}x \tan x +\frac{3}{8}(\sec x \tan x+ \ln| \sec x + tan x |)+C}

Print Friendly, PDF & Email
Post Views: 892

Be the first to comment

Leave a Reply

Your email address will not be published.


*