Perimeter of a polygon:
The perimeter of a Polygon is the sum of the lengths of all sides of the polygon.
Perimeter of a triangle:
-For a scalene Triangle of sides ![]() ,
, ![]() and
and ![]() , the perimeter
, the perimeter ![]() is:
is:
![]()
-For Isoceles Triangle of Equal side ![]() and base
and base ![]() :
:
![]()
-For an Equilateral Triangle of side ![]() :
:
![]()
-Perimeter of Quadrilaterals:
-For a quadrilateral of sides ![]() ,
,![]() ,
,![]() and
and ![]() :
:
![]()
-For a Rectangle of Length ![]() and width
and width ![]()
![]()
Or
![]()
-For a Square(or Rhombus) of side ![]() :
:
![]()
-For a Parallelogram of parallel equal sides ![]() and parallel equal sides
and parallel equal sides ![]() :
:
![]()
Or
![]()
Areas of Circles and Polygons:
A triangular region consists of a triangle and its interior while a circular region is one of a circle and its interior.
The area of a triangle can be calculated using methods depending on information we have:
For a scalene triangle:
-Taking any altitude (as height, h) and its opposite side (as base, b).
The Area is:
![]()
We can also write:
![]()
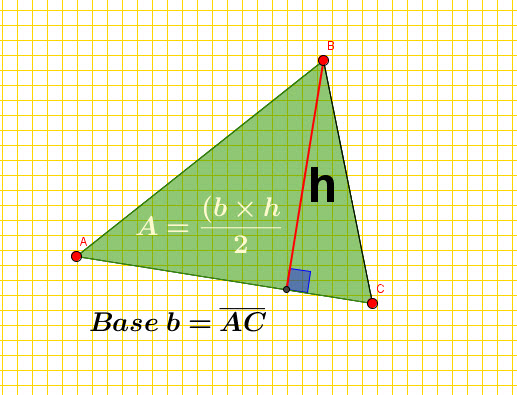
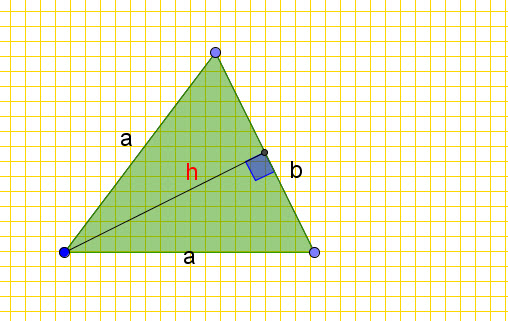
For an isosceles triangle, given the three sides: a,a and b
We can calculate ![]()
![]()
Finally:
![]()
And the area of the triangle:
![]()
If we use the Heron formula, we find the same formula
![]()
In our situation ![]() and
and ![]()
That means ![]()
![]()
![]()
![]()
We get the following area:
![]()
![]()
Finally:
![]()
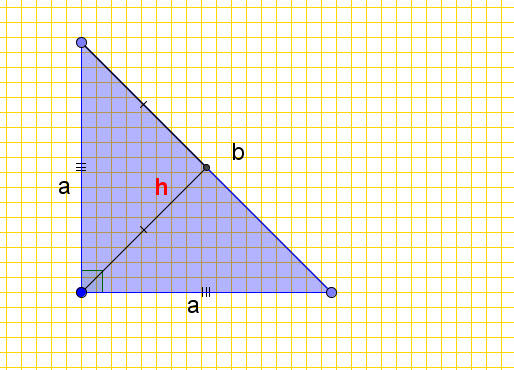
Special isosceles and right triangle:
In this situation we have :
![]()
Without going far, we can notice that each of the right triangle legs can be the base (a) or the height (a):
![]()
![]()
Using b:
![]()
This can be found from the previous formula as well.
Equilateral Triangle
We have the nomrmal formula:
![]()
![]()
![]()
![]()
![]()
Now for the area we have ![]()
![]()
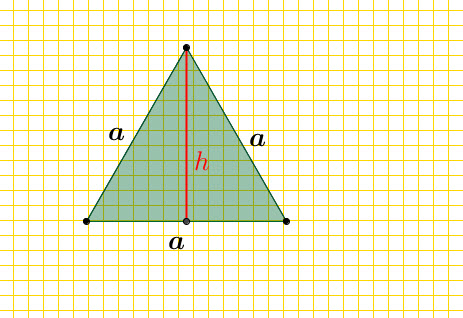
finally:
![]()
Area Triangle using angles:
We can see that:
![]()
![]()
So: ![]()
The Area:
![]()
We can find all three relationships:
![]()
![]()
![]()
Circumference of a circle
The Circumference ![]() of a circle is as follows:
of a circle is as follows:
![]()
where ![]() is the radius of the circle.
is the radius of the circle.
Since the diameter ![]() , we can write:
, we can write:
![]()

Areas and circumferences of circular figures can be calculated without replacing ![]() with its value. That means in terms of
with its value. That means in terms of ![]() .
.
Length of an arc:
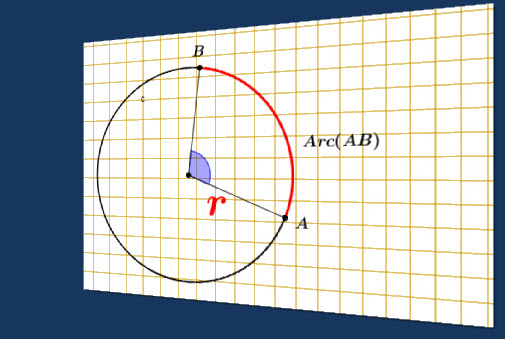
The length of an arc of a circle is related to the central angle ![]() that intercepts it.
that intercepts it.
If ![]() is in
is in ![]() , we have:
, we have:
![]()
For an angle in ![]() , we use the proportion to the circumference:
, we use the proportion to the circumference:
The length of ![]()
This is the same as:
![]()
Area of a Circle of radius r:
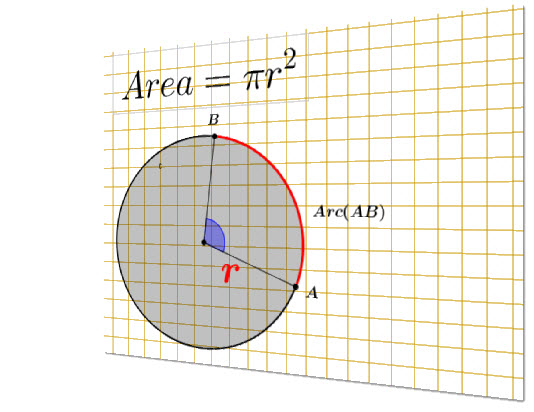
If ![]() is the radius of a circle, the
is the radius of a circle, the ![]() is:
is:
![]()
This formula is widely used in geometry and other fields.
Area of a Sector AOB of a circle:

If ![]() is the radius of a circle,
is the radius of a circle, ![]() the central angle (or intercepted arc) in
the central angle (or intercepted arc) in ![]() , the
, the ![]() of
of ![]() is:
is:
![]()
We simplify and get:
![]()
If ![]() the central angle (or intercepted arc) is in
the central angle (or intercepted arc) is in ![]() :
:
![]()
This is a simple conversion of ![]() radians above.
radians above. ![]()
Area of a segment of a circle
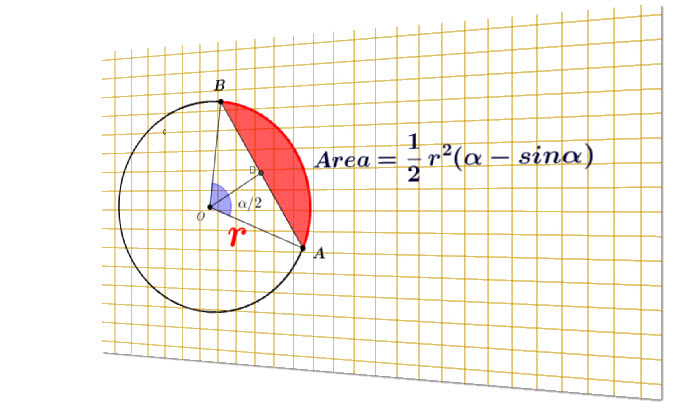
This can be approached 2 ways:
-Area of the sector minus area of isosceles triangle ![]()
-By deriving the formula.
The central angle’s bisector bisects the chord AB at D.
We get
![]()
But ![]()
![]()
OD is the altitude:
![]()
![]()
![]()
![]()
![]()
But we know that:
![]()
That returns:
![]()
The Area of ![]() is:
is:
![]()
We plug in:
![]()
Finally:
![]()
For sector AOB:
![]()
We take the difference to get the area of the segment:
![]()
![]()
Al-Kashi, Heron,Bretschneider’s, Brahmagupta’s and Coolidge formulas
The Bretscheiner’s formula helps find the area of a non-cyclic quadrilateral, that cannot be inscribed in a circle,
using only side lengths and possibly one angle measure or one diagonal length.
After the Bretschneider’s formula, we’ll simplify the quadrilateral to make it cyclic. We’ll get the Brahmagupta’s formula.
With the cyclic quadrilateral the product of the diagonals ![]() and
and ![]() is
is ![]() and opposite angles are supplementary.
and opposite angles are supplementary.
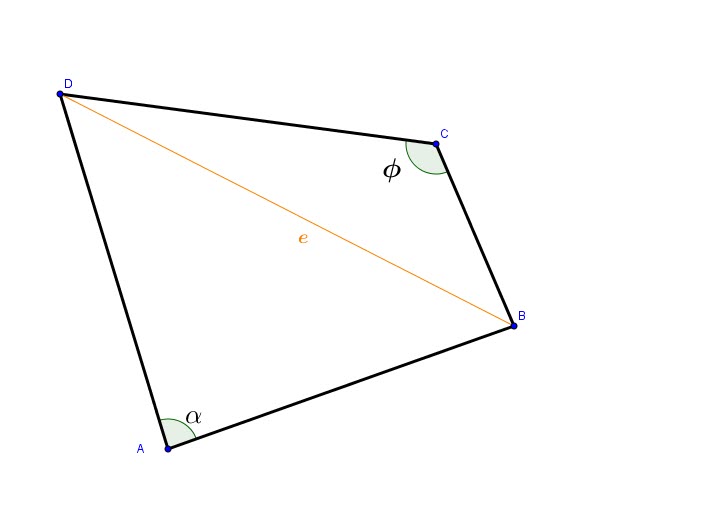
Let ![]() be area.
be area.
From the figure, we notice ![]()
![]()
![]()
Squaring both sides:
![]()
![]()
![]()
Now let’s check 2 values of diagonal ![]() :
:
![]()
![]()
![]()
![]()
![]()
Taking the square:
![]()
![]()
Adding ![]() and
and ![]() :
:
![]()
![]()
![]()
![]()
![]() Injected 2 in the last term.
Injected 2 in the last term.
![]()
To the same denominator and multiplying both sides by 4:
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()

If we call the semi-perimeter ![]()
We have the final Bretscheiner’s formula:
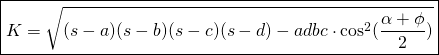
Brahmagupta’s formula
The steps remain the same but this time since the quadrilateral is ![]() we know that the two opposite angles are supplementary.
we know that the two opposite angles are supplementary.
![]()
We get from the Bretscheiner’s formula
![]()
Heron’s formula
The fourth side goes away and we get a triangle. From Brahmagupta’s formula:
![]()
In depth explanation:
From Al-Kashi to Bretshneider via Heron and Brahmagupta, we are going to go through some of their work:
Al-kashi is simply the Law of Cosines:
Law of cosines:
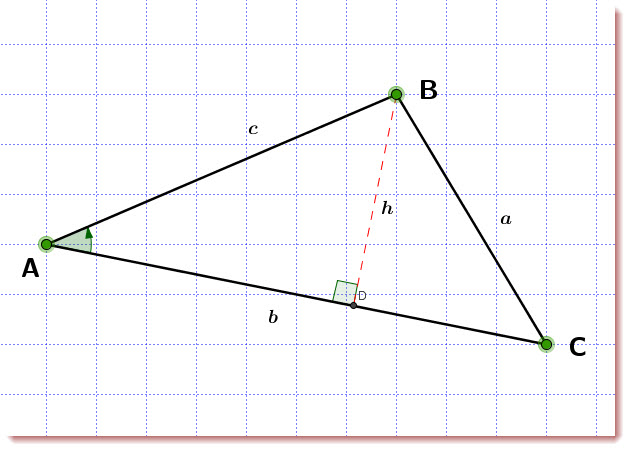
From what we know:
![]()
We have two right triangles: ![]() and
and ![]()
Using the Pythagorean Theorem:
![]()
Also:
![]()
![]()
But :
![]()
This means:

![]()
![]()
![]()
![]()
We know from above: ![]()
![]()
Finally we have the ![]() :
:
![]()
![]()
![]()
Heron Formula:
This one is widely used. We use it when we have only sides and we want to find the Area of the triangle
Let![]() be area and
be area and ![]() the semi-perimeter.
the semi-perimeter.
![]() .
.
We should remember this because we’ll need it in the following paragraphs.
![]()
![]()
But we know that:
![]()
![]()
![]()
![]()
![]()
This is a difference of squares, we can factor:
![]()
![]()
![]()
![]()
![]()
![]()
![]()
This is the Heron formula:
![]()
The Bretschneider formula:
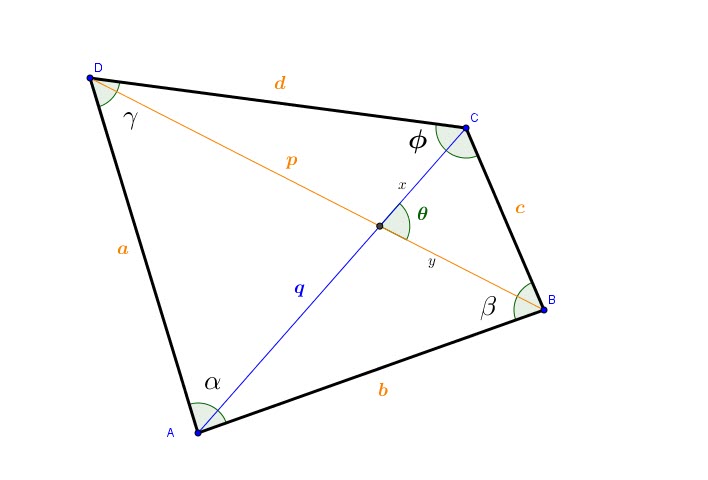
We will demonstrate the following forms of the Area ![]() per BRETSCHNEIDER
per BRETSCHNEIDER
![]()
![]()
![]()
We split the diagonal ![]() into
into ![]() and
and ![]()
The same way for ![]() :
: ![]() and
and ![]()
We express the area of the four triangles:
![]()
![]()
![]()
![]()
Now we add all four areas:
![]()
![]()
After simplication:
![]()
The first form:
![]()
Now the second form:
From the figure:
![]()
![]()
![]()
![]()
We expand and replace terms in ![]() by
by ![]()
![]()
Now we move to the ![]()
![]()
![]()
We expand and replace terms in ![]() by
by ![]()
![]()
Now we move to the ![]()
![]()
![]()
![]()
Let’s add as follows:
![]()
![]()
![]()
After simplification we get:
![]()
But:
![]()
We plug in ![]()
![]()
Second Form:
![]()
Third form:
From the sequences above:
![]()
But: ![]()
![]()
![]()
![]()
![]()
This is the actual BRETSCHNEIDER formula:
![]()
According to wiki, the following work is credited to COOLIDGE in 1939:
Let ![]() be area.
be area.
From the figure, we notice ![]()
![]()
![]()
Squaring both sides:
![]()
![]()
![]()
Now let’s check 2 values of diagonal ![]() :
:
![]()
![]()
![]()
![]()
![]()
Taking the square:
![]()
![]()
Adding ![]() and
and ![]() :
:
![]()
![]()
![]()
![]()
![]()
![]() Injected 2 in the last term.
Injected 2 in the last term.
![]()
To the same denominator and multiplying both sides by 4:
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()

If we call the semi-perimeter ![]()
We have the final COOLIDGE formula:


Be the first to comment